Banking of Roads:
When a car moves along a curved road, it requires some centripetal force. During the turn, the wheels of the car have a tendency to leave the curved path and regain the straight line path. The frictional force between the wheels and the road opposes this tendency of the wheels. Therefore, the frictional force acting towards the centre of circular track provides the necessary centripetal force.
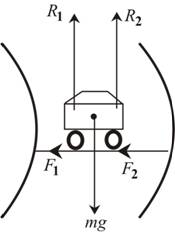
Figure:12.a
When the road is levelled, the normal reaction forces of the road equal
to the weight of the car.
$R_{1}+R_{2}=mg$
If $F_{1}$and $F_{2}$are the frictional forces, then
$F_{1}=\mu R_{1}$and $F_{2}=\mu R_{2}$
Where $\mu $ is the coefficient of friction between tyres and road.
While rounding the curve, the centripetal force required is $mv^{2}/r$.
Where $v$ is the velocity of the car.
As the centripetal force is provided by the friction, therefore,
$mv^{2}/r$ $\le $ ($F_{1}+F_{2}$)
Or, $mv^{2}/r$ $\le $ $\mu $($R_{1}+R_{2}$)
Or, $mv^{2}/r$ $\le $ $\mu $$mg$
Or, $v=\sqrt{\mu rg}$
So, the maximum velocity with which a vehicle can go round a level curve without skidding is $v=\sqrt{\mu rg}$.
For a curved track, the sufficient centripetal force is managed by raising the outer edge of the track a little above the inner edge. It is called banking of the roads.
Suppose, a car of weight $Mg$ moving round a curved path of radius $r$ with speed $V$on the road banked through angle $\theta $. If $OA$ is banked road and $OX$ is the horizontal line, then angle $AOX=\theta $.
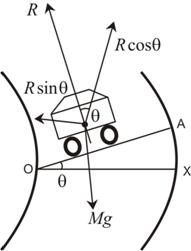
Figure:12.b
Following are the forces to be considered.
- $Mg$ acting vertically downwards
- Reaction $R$ of the ground acting along normal to the banked road $OA$ in upward direction
- $R cos \theta $ will balance $Mg$.
- $R sin \theta $will provide necessary centripetal force to the vehicle.
Therefore,
$R cos \theta =$ $Mg$, $R sin \theta =Mv^{2}/r$
$R sin \theta /R cos \theta =v^{2}/rg $
Or, Or, $tan \theta =v^{2}/rg$ ………………(1)
Knowing $v$ and $r$, the angle of banking $\theta $can be calculated,
If $h$ is the height $AX$ of outer edge of the road, then
$OX=\sqrt{b^{2}-h^{2}}$
$tan \theta =h/\sqrt{b^{2}-h^{2}}$ …………………(2)
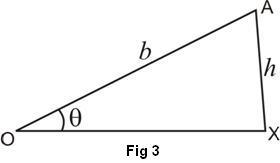
Figure:12.c
From equations (1) and (2) we get,
$tan \theta =h/\sqrt{b^{2}-h^{2}}=v^{2}/rg$
As $h << b$, then, we get, $tan \theta =h/\sqrt{b^{2}}=h/b=$$v^{2}/rg$
Points to remember:
- The angle of banking is an angle at which a vehicle is inclined about its longitudinal axis with respect to the plane of its curved path.
- If the frictional force is not strong enough, the vehicle will skid.
- Roads are banked because of the inertia of wheels and vehicles driving on the road.
