Derivative introduced as rate of change both as that of distance function and geometrically
A rate can be explained as the comparison between two quantities of different kinds. A common rate that we use as a physical quantity is a speed. Speed is defined as the rate of distance traveled per unit of time. Also, recall from physics that distance is a function of time. So, we can draw a graph for distance over time. In this case, let distance be the function f of time, x. The average rate of change of the function f over the interval [a, b] can be written as:
$\frac{\Delta f}{\Delta x}=\frac{f(b)-f(a)}{b-a}$
For a line, the rate (or slope) is the same at every point on the line. The fraction Δf/Δx represents a velocity because it is measured in units of distance over time. But this is only an average velocity between two points on the graph. This is because we consider the distances and times of two points. The length of the time intervals we consider is Δx. To make better approximations, we can take smaller and smaller time intervals. In the language of calculus, we take the limit of the slope as Δx approaches 0 and calculate the instantaneous velocity at an instant A. This can be shown as:
$speed=\lim _{x?0}\frac{\Delta f}{\Delta x}$
The same definition of the derivative can be arrived at using the geometric intuition. For graphs other than lines, the rate at which the graph rises or falls changes from point to point which can be calculated as the slope of the linear graph.
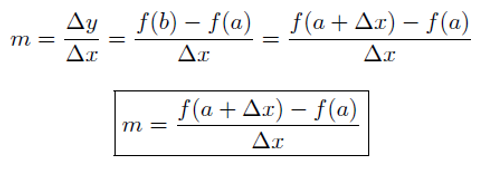
To determine the rate at which a quadratic graph rises or falls at a single point, we can find the slope of the tangent line to the point. The instantaneous rate of change of f(x) at x is defined by taking the limit of the average rates of change of ‘f’ over the intervals [x, x+∆x], as ∆x approaches 0. The derivative is the slope of the tangent line to a graph f(x) and is usually denoted f’(x). The slope of the secant line through (x, f(x)) and (x+∆x, f(x+∆x)) is the same as the average rate of change of f over the interval [x, x+∆x]. The slope of the tangent line through (x, f(x)) is the same as the instantaneous rate of change of f at the point x, or the derivative. The slope of the tangent, or derivative, depends on the position of the point (x, f(x) on the curve, and therefore on the choice of x. Therefore, the derivative is a function of x, and that is why we write it as f'(x).
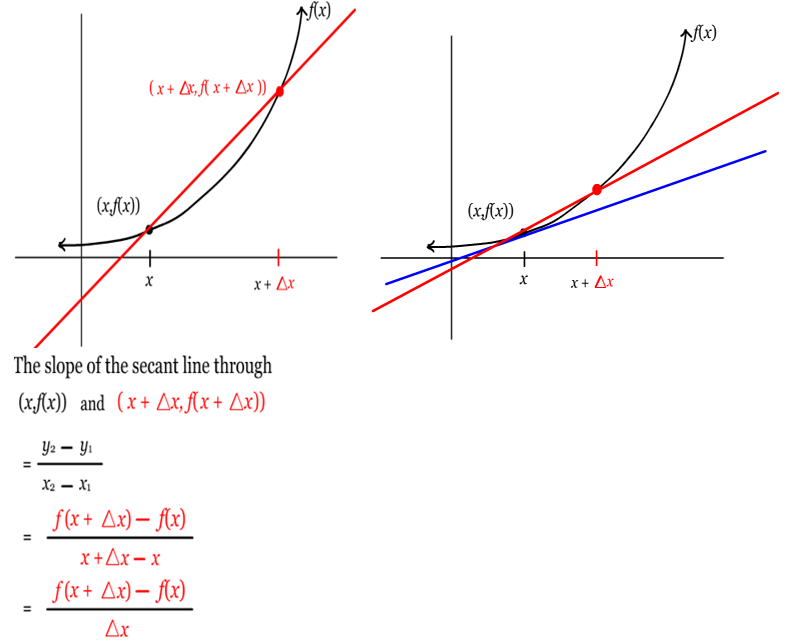
The smaller the ∆x, the more the secant and tangent lines resemble each other.The derivative f'(x) of the function f(x) is the slope of the tangent at the point (x, f(x)).We define the derivative of the function f at the point x0 as shown below:
![]()
The symbol on the left of the above equation is read “f prime of x zero”. Now, the point x0 that we originally chose was arbitrary. We can do the same for any other point. So, what we get really is a function of x.
