Geometric Definition of a Parabola: The collection of all the points P(x,y) in a plane at the same distance from a fixed point, the focus, as they are from a fixed line called the directrix. Note that “p” represents the distance from the focus to the vertex or the distance from the vertex to the directrix on a parabola.Note that, the vertex is the midpoint from the focus to the directrix.
Point Focus = Point Directrix
PF = PD
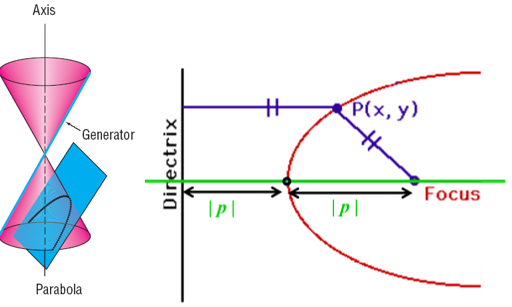
The parabola has an axis of symmetry that intersects the parabola at its vertex.The distance from the vertex to the focus and from the directrix to the vertex is | p |as shown in the graph above.
Consider the figure below where the focus of a parabola is the point F(0, p), the axis of symmetry must be vertical and the directrix has the equation y = –p. The figure below illustrates the case p>0:
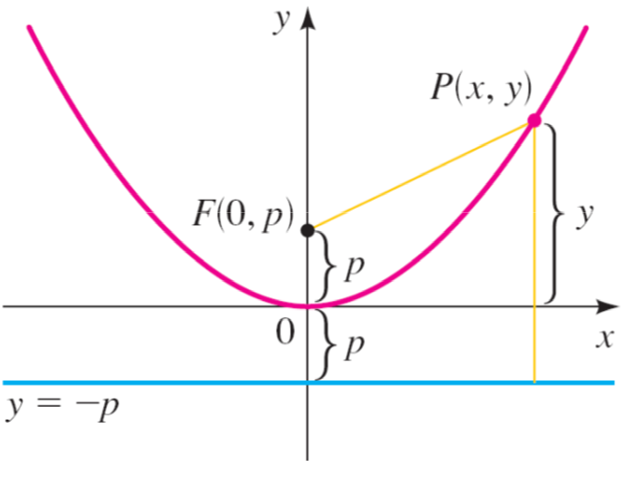
If P(x, y) is any point on the parabola, then the distance from P to F using the Distance Formula can be written as:
Note that, the distance from P to the directrix is: | y – (–p) | = | y +p |
By the definition of a parabola, equating these two equations give the equation for the parabola:
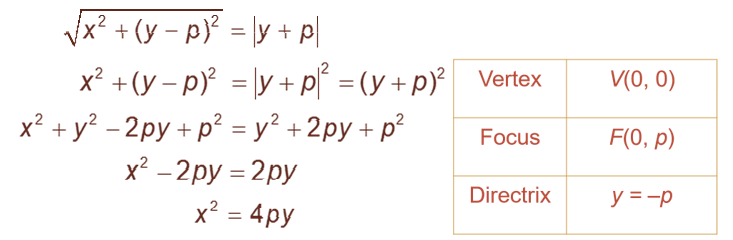
If p > 0, then the parabola opens upward and itp < 0, the parabola opens downward. When x is replaced by –x, the equation remains unchanged. So, the graph is symmetric about the y-axis.
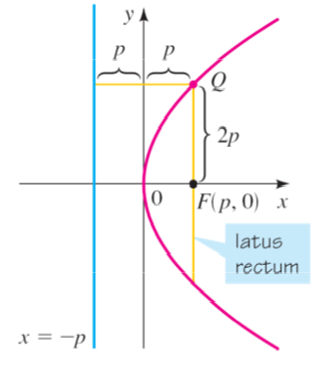
Consider the figure above where the line segment that runs through the focus perpendicular to the axis—with endpoints on the parabola—is called the latus rectum which is the focal diameter of the parabola. From the figure above, we can see that the distance from an endpoint Q of the latus rectum to the directrix is |2p|.Therefore, the distance from Q to F must be | 2p | by the definition of a parabola.Hence, the focal diameter is | 4p |.
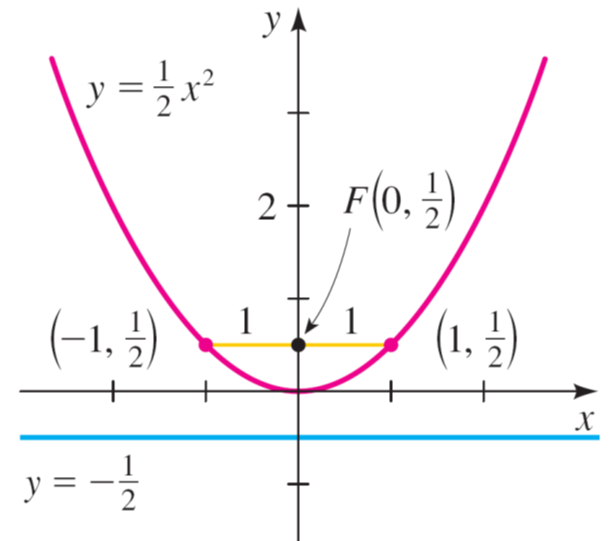
Example: Find the focus, directrix, and focal diameter of the parabola y = ½x2, and sketch its graph.
We put the equation in the form x2 = 4py.
y = ½x2
x2 = 2y
We see that 4p = 2.So, the focal diameter is 2
Solving for p gives p = ½.Therefore, the focus, F is (0, ½) and the directrix is y = –½.
The focal diameter is 2. This means that the latus rectum extends 1 unit to the left and 1 unit to the right of the focus.
Parabolas have an important property that makes them useful as reflectors for lamps and telescopes as well as architectural structures such as suspension bridges.
