Introduction to Three–dimensional Geometry: Coordinate axes and coordinate planes in three dimensions
To locate a point in a plane, two numbers are needed. Recall that any point in the plane can be represented as an ordered pair (a, b) of real numbers, where a is the x-coordinate and b is the y-coordinate. For this reason, a plane is called two-dimensional. Alternatively, to locate a point in space, three numbers are necessary. We represent any point in space by an ordered triplet (a, b, c) of real numbers.For this purpose, we first choose a fixed point O (the origin) and three lines passing through O that are perpendicular to each other. These lines are called the coordinate axes and labelled the x-axis, y-axis, and z-axis.Usually, we think of the x- and y-axes as being horizontal and the z-axis as being vertical, and we draw the orientation of the axes as shown in the figure below:
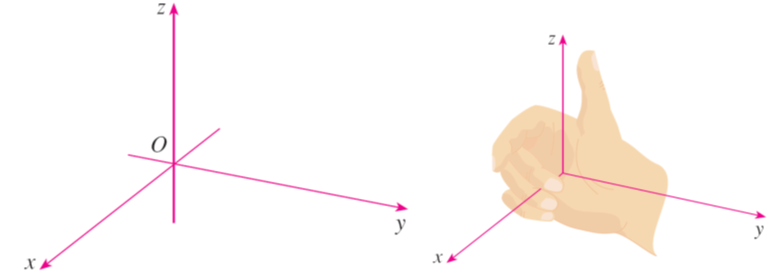
The direction of the z-axis is decided by using the right-hand rule. When you fold the fingers of your right hand around the z-axis in the direction of a 90 anticlockwise rotation from the +ivex-axis to the +ivey-axis, then your thumb points in the +ive direction of the z-axis.
The three coordinate axes determine the three coordinate planes illustrated in the figure below:
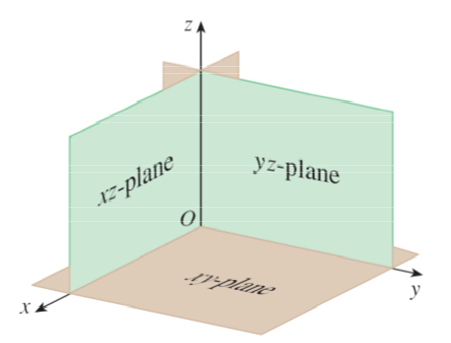
The figure shows that the xy-plane is the plane that contains the x- and y-axes; yz-plane: y- and z-axes; and xz-plane: x- and z-axes. These three coordinate planes divide three-dimensional space into eight sections called octants. The first octant, in the foreground, is most often used and it is determined by the positive axes.The others are shown in the figure below:
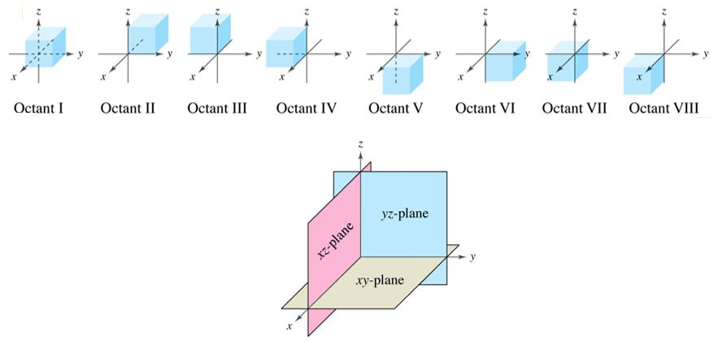
| Number | Name | x | y | z |
| I | Top-front-right | + | + | + |
| II | Top-back-right | – | + | + |
| III | Top-back-left | – | – | + |
| IV | Top-front-left | + | – | + |
| V | Bottom-front-right | + | + | – |
| VI | Bottom-back-right | – | + | – |
| VII | Bottom -back-left | – | – | – |
| VIII | Bottom-front-left | + | – | – |
Every plane can be explained by using a linear equation which is written as:
![]()
Here A, B, and C≠ 0 to ensure a 3D plane. Note that, the first-degree equation in three variables represents a plane that is placed in Cartesian space.
Example: Sketch the graph of 12x +15y + 20z = 60
This is a first-degree equation, therefore the graph forms a plane and not a point. We need three points to determine a plane.
Divide both sides by 60:
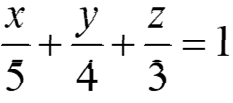
It’s now easy to see that the following points are on the plane: (5, 0, 0), (0, 4, 0) and (0, 0, 3)
