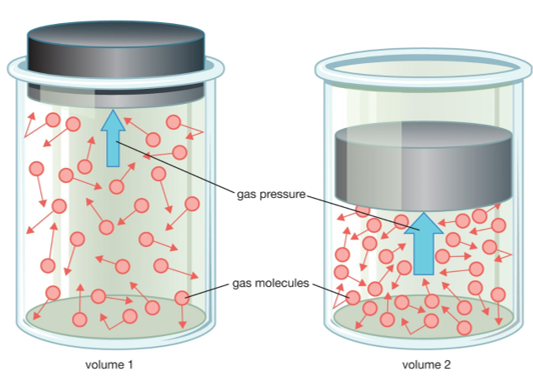
All of the thermodynamic properties of an ideal gas are summed up in its equation of state, which determines the relationship between its pressure, volume, and temperature. Unfortunately, classical thermodynamics is unable to tell us what this equation of state is from first principles. In fact, classical thermodynamics will not be able to tell us anything from the very first principles. We always have to provide some information to begin with before classical thermodynamics can generate any new results. This initial information may come from statistical physics (i.e., from our knowledge of the microscopic structure of the system under consideration), but, more usually, it is entirely empirical in nature (i.e., it is the result of experiments). Of course, the ideal gas law was first discovered empirically by Robert Boyle, but, nowadays, we can justify it from statistical arguments. That the number of accessible states of a monotonic ideal gas varies like: \mit\Omega \propto V^N \chi (E),
Ideal Gas Equation of Stats
For perfect gas, the ideal gas equation is
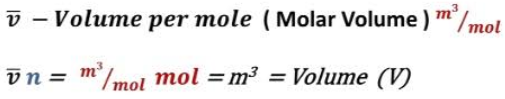
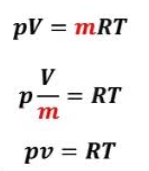
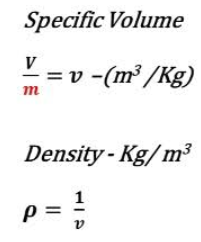

Where $N$ is the number of atoms, and $\chi (E) $ depends only on the energy of the gas (and is independent of the volume). We obtained this result by integrating over the volume of accessible phase-space. Since the energy of an ideal gas is independent of the particle coordinates (because there are no interatomic forces), the integrals over the coordinates just reduced to $N$ simultaneous volume integrals, giving the $V^N$ factor in the above expression. The integrals over the particle momenta were more complicated, but were clearly completely independent of $V$, giving the $\chi (E) $ factor in the above expression. A statistical rule which tells us that for an ideal gas, the only external parameter is the volume, and its conjugate force is the pressure (since ${\mathchar’26\mskip-12mud} W =p\, dV$).
However, $N= \nu \,N_A$, where $\nu$ is the number of moles, and $N_A$ is Avogadro’s number. Also, $k\,N_A = R$, where $R$ is the ideal gas constant. This allows us to write the equation of state in its usual form
p\, V = \nu\, R\, T.
The derivation mentioned above of the ideal gas equation of state is rather more elegant. It is certainly far easier to obtain the equation of state in this manner than to treat the atoms which make up the gas as little billiard balls that continually bounce off the walls of a container. The latter derivation is quite hard to perform correctly because it is necessary to get average overall attainable directions of atomic motion. It is clear, from the derivation mentioned above, that the crucial element required to obtain the ideal gas equation of state is the absence of interatomic forces. This automatically gives rise to a variation of the number of accessible states with $E$ and $V$ of the form, which, in turn, implies the ideal gas law. So, the ideal gas law should also apply to polyatomic gases with no interatomic forces.
