Exponential and logarithmic functions

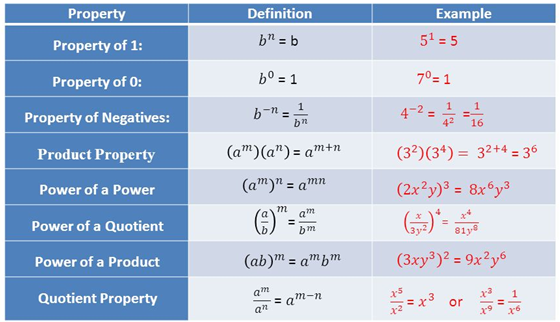
The function defined by f(x) = b^x; (b>0), b≠1) is called an exponential function with base b and exponent x.Here, the domain of f can be explained as a set of all real numbers. Let m and n be positive numbers and let a and b be real numbers. Then,

The exponential function y = b^x (b> 0, b ≠ 1) is associated with the following properties:

• 1. The domain is (–∞, ∞).
  2. Range is (0, ∞).
  3. The graph passes through the point (0, 1)
  4. Continuous on (–∞, ∞).
  5. Increasing function on all real numbers (–∞, ∞); b>1 and a decreasing function on (–∞, ∞); b< 1, such that b ≠ 1.

Exponential functions to the base e play a prominent role in theoretical and applied problems such as growth and ready of a natural phenomenon. Here e is an irrational number whose value is 2.7182818…. It can be shown that

$e=\lim _{m\to \infty }(1+\frac{1}{m})^{m}$

You can write an exponential equation as a logarithmic equation and vice versa in the following format.

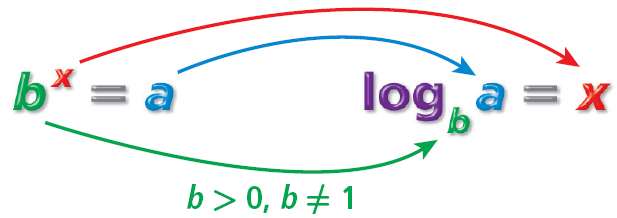
The function defined by f(x) = log_bx; (b>0, b≠1) is called the logarithmic function with base b. The domain of f is this case is a set of all positive numbers and not real numbers. The following properties of logarithmic functions show M and N as positive real numbers, b ≠ 1, such that p and x are real numbers.

The function log_ba= x is read as “the log base b of a is x.” Notice that the log is the exponent.A logarithm containing base 10 is defined as a common logarithm. A logarithm containing base e is defined as a natural logarithm. When no base is mentioned for a logarithmic function, the base is assumed to be 10. For example, log 5 = log₁₀5.

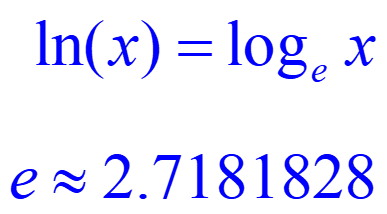
Properties relating e^x and ln x:

1. e^lnx = x (x> 0)
2. ln e^x = x (x = real number)

Logarithms are the inverses of exponents, the inverse of an exponential function, such as y = 2^x, is a logarithmic function, such as y = log₂x.The domain of y = 2^x is all real numbers (R), and the range is {y|y> 0}. The domain of y = log₂x is {x|x> 0}, and the range is all real numbers (R).

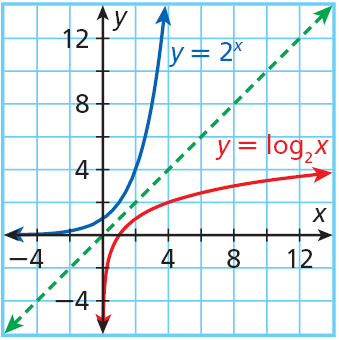
The logarithmic function y = log_bx (b > 0, b ≠ 1) is true for the following properties:

• 1. Domain is (0, ∞).
  2. The range is (–∞, ∞).
  3. The graph passes through the point (1, 0).
  4. Continuous on (0, ∞).
  5. Increasing fucntion on the range (0, ∞); b > 1 and a decreasing function on (0, ∞); b < 1, such that b ≠ 1.

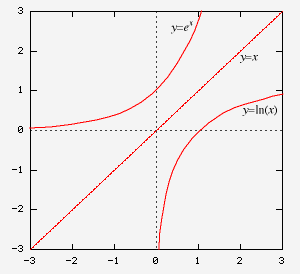
Example: Sketch the graph of y = ln x and show its relationship with y = x.

Solution: The required graph is the mirror image of the graph of y = e^x with respect to the line y = x: