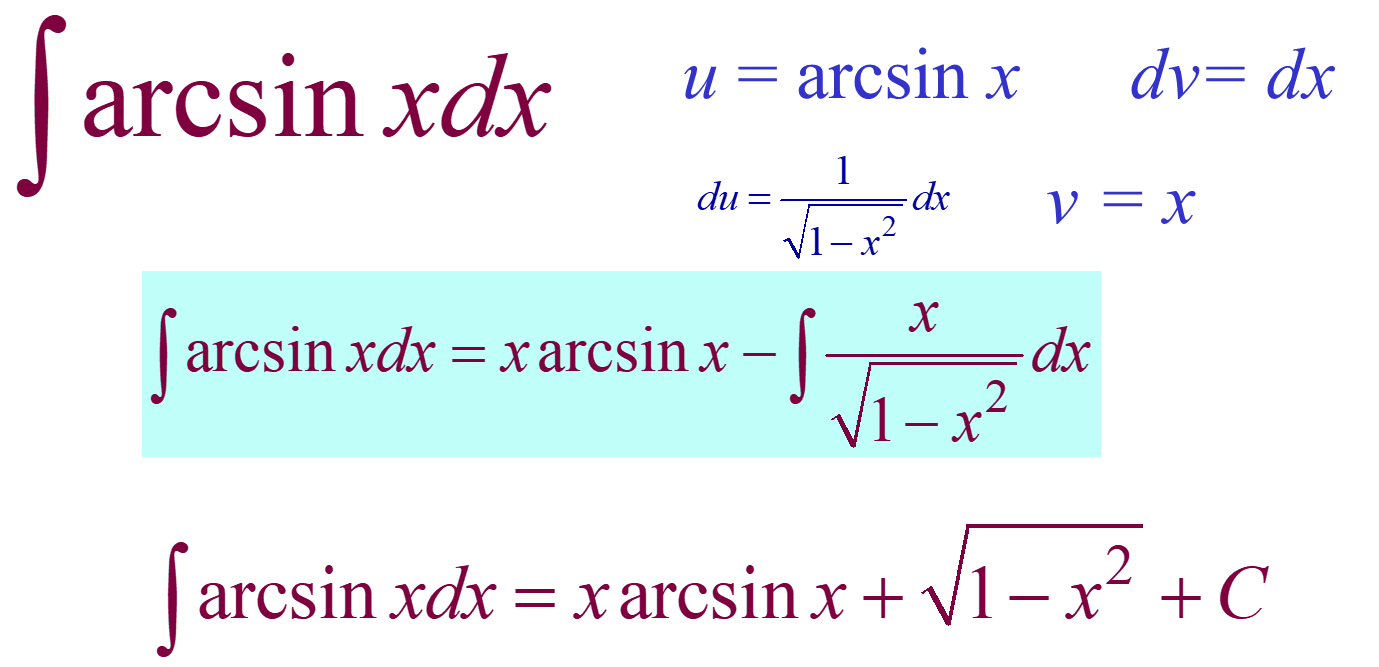Integration of a variety of functions by substitution, by partial fractions and by parts
Integration by substitution
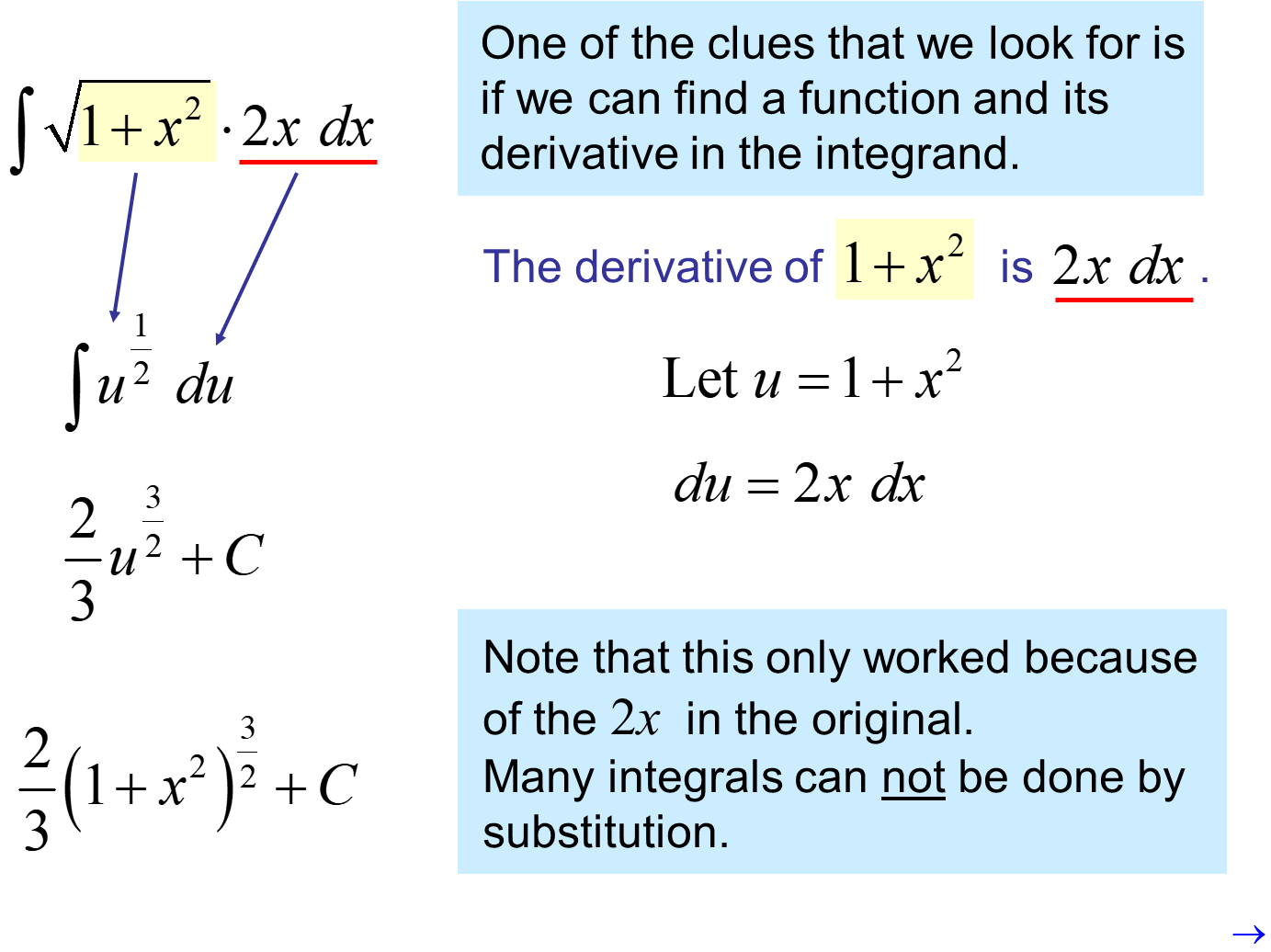
Consider the function:
![]()
To find the integral of a complex function such as this, we introduce a new variable. We change the function of x from the variable x to a new variable u.Suppose that we let u be the quantity under the root sign in the equation: u = 1 + x2 such that du = 2x dx. The dx for an integral is a differential such that,2x dx ,hence:
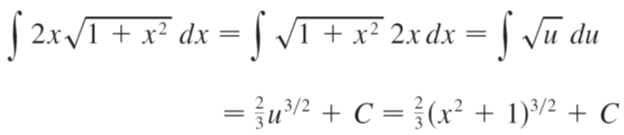
But now we can check that we have the correct answer:
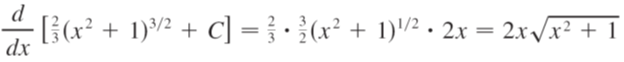
In general, this method works with integral in the form f (g(x))g(x) dx. Observe that if F = f,then F(g(x))g(x) dx = F (g(x)) + C, because, by the Chain Rule, $\frac{d}{dx}$ [F (g(x))] = F(g(x))g(x) . If we make the “change of variable” or “substitution” u = g(x),
F(g(x))g(x) dx = F (g(x)) + C = F(u) + C = F(u) du
or, writing F = f, we getf (g(x))g(x) dx =f (u) du
Thus the Substitution Rule explains that It is allowed to operate with dx and du after integral signs as if they were differentials.
Example: consider the following equation:
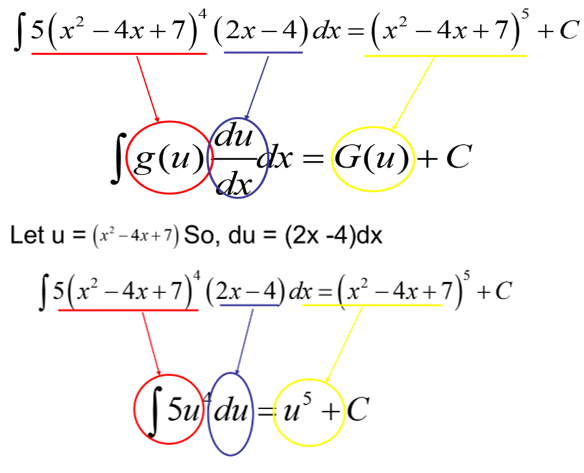
Example:Find the indefinite integral
Integration by partial fraction
We can integrate any rational function (a ratio of polynomials) by showing it as a sum of simpler fractions, called partial fractions,which can be integrated easily.Hence, by taking the fractions 2/(x – 1) and 1/(x + 2) to a common denominator we obtain:
![]()
If we now reverse the procedure on the right side of this equation:
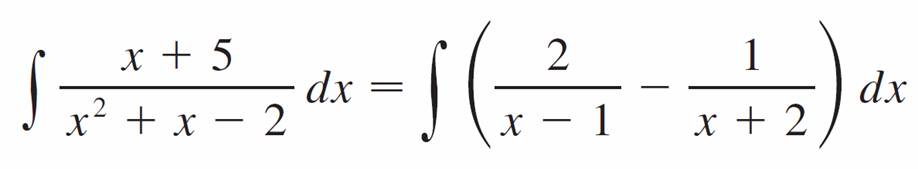
= 2 ln | x – 1 | – ln | x + 2 | + C
To understand further, let’s consider a rational function:
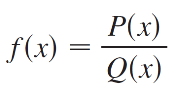
where P and Q are denoted as polynomial functions. It’s possible to express f as a sum of simpler fractions provided that the degree of P is less than the degree of Q. Such a rational function is called a proper function.
Recall that ifP (x)= anxn+ an– 1xn – 1 + . . . + a1x + a0, where an ≠ 0, then the degree of P is n and we write deg(P) = n.If f is improper, that is, deg(P) deg(Q), then we must take the preliminary step of dividing Q into P (by long division) until a remainder R (x) is obtained such that deg(R) < deg(Q).
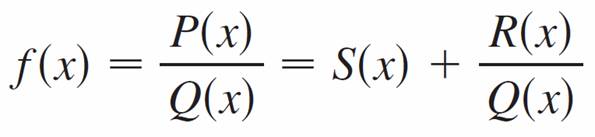
where S and R are also polynomial functions.
Example: Find 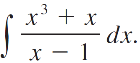
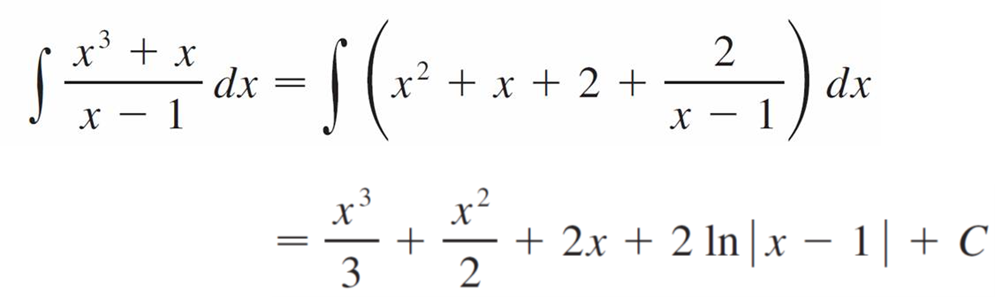
Example: Evaluate 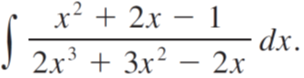
We factor the denominator as
2x3 + 3x2 – 2x =x(2x2 + 3x – 2)
= x(2x – 1)(x + 2)
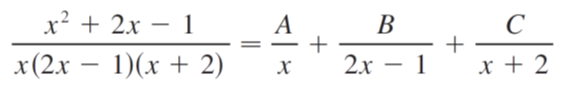
To find A, B, and C, we multiply both sides of this equation by the product of the denominators, x(2x – 1)(x + 2).
We obtain:
x2 + 2x – 1 = A(2x – 1)(x + 2) + Bx(x + 2) + Cx(2x – 1)
x2 + 2x – 1 = (2A +B + 2C)x2 + (3A + 2B –C)x – 2A
Therefore, we obtain 3 equations:
- 2A + B + 2C = 1
- 3A + 2B – C = 2
- –2A = –1
Solving, we get, A = 1/2B = 1/5 and C = -1/10 and so
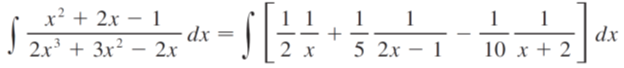
![]()
In integrating the mid term using substitution u = 2x – 1, which gives du = 2 dx and dx = ½ du.
Integration by parts
It is a rule that transforms the integral of products of functions into other functions. When these functions are not related we can use integration by parts to find the integral of the function. This mode of integration is obtained from product rule:
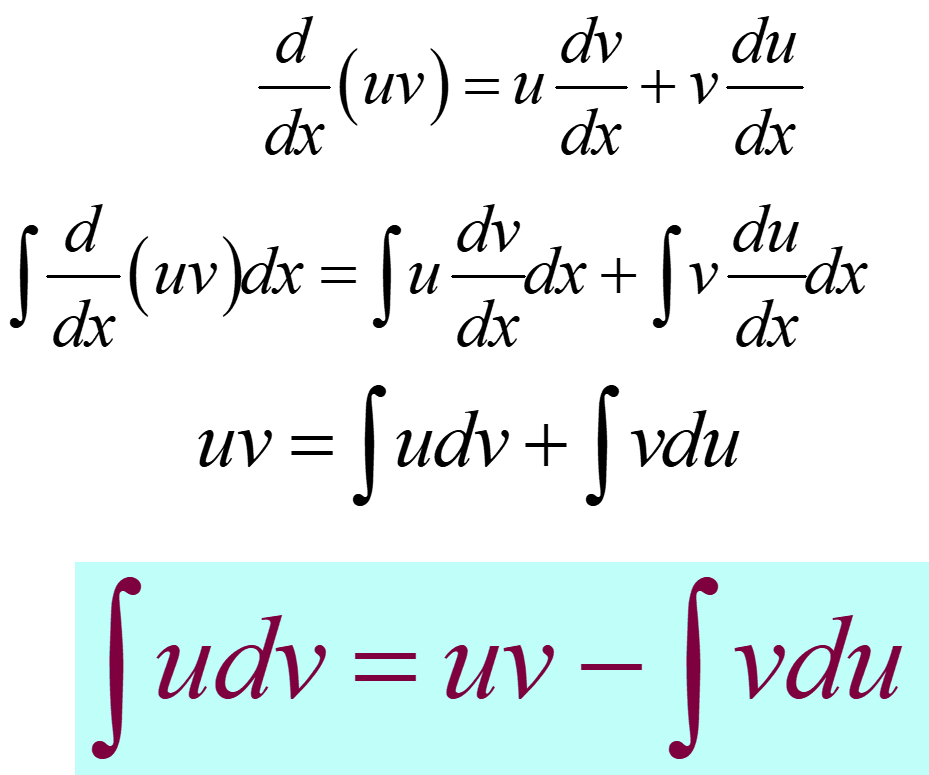
Let dv be the most complicated part of the original integrand which fits a basic integration rule that includes dx. Then u indicates the remaining factors.
Example:
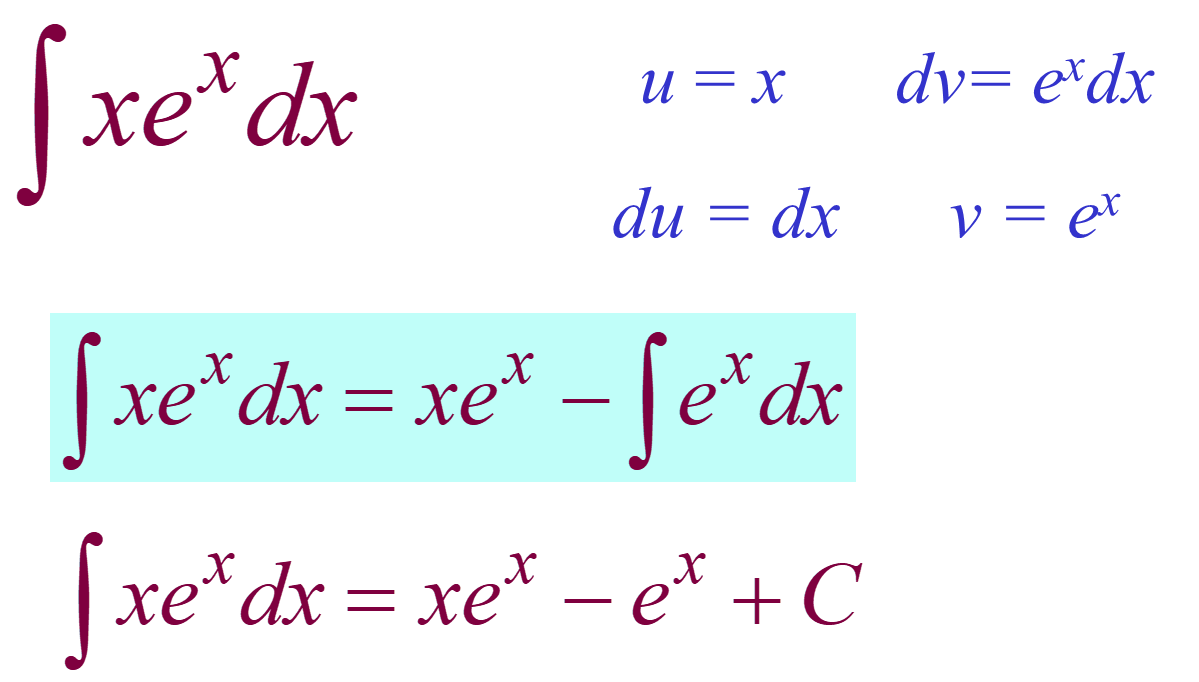
Example: