Projectile motion is a type of motion in which an object called a projectile is thrown or projected. It is an example of a two-dimensional motion with constant acceleration.
The projectile is thrown with some initial velocity near the earth’s surface, and it moves along a curved path under the influence of gravity.
There are two simultaneous motion in mutually perpendicular directions which are completely independent of each other. The diagram is shown below.
Acceleration of the projectile: When a projectile is thrown in the air with some velocity, the only force acting on it during its time in the air is because of acceleration due to gravity (g). This acceleration acts vertically downward. There is no acceleration in the horizontal direction i.e. the horizontal direction remains constant.
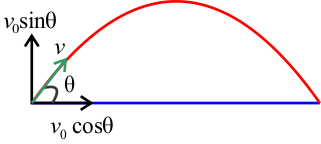
Figure:8.a
Suppose a projectile is launched with velocity $u$making an angle $\theta $with the horizontal.
The point O is called the point of projection; θ is the angle of projection.
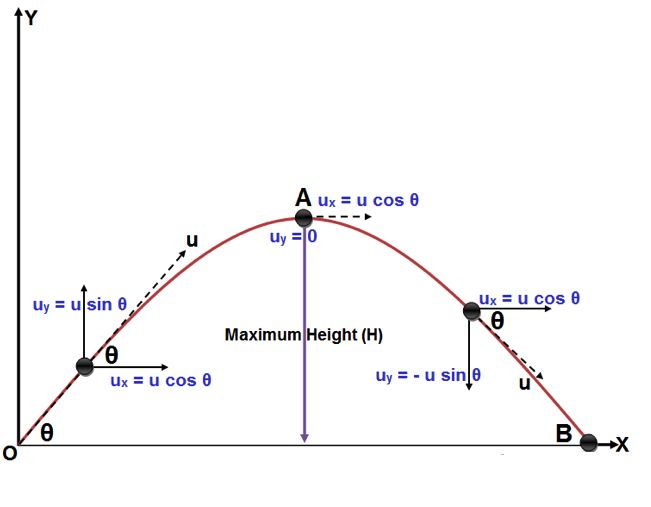
Figure:8.b
Let us find different parameters related to projectile motion using kinematic equations.
Component of velocity along X-axis, $u_{x}=u cos\theta $
Horizontal acceleration, $a_{x}=0$
Component of velocity along Y-axis, $u_{y}=u sin\theta $
Vertical acceleration, $a_{y}=-g$
Time of maximum height:
Let us consider the motion along Y-direction:
Let us first consider the motion of the projectile from point O to A.
Initial velocity along Y-direction, $u_{y}=u sin\theta $and acceleration, $a_{y}=-g$
If B is the maximum height that the projectile has reached and after that it falls, so final velocity at B along Y-direction, $v_{y}=$0
If t is the time taken to travel from O to A, then using the equation of motion $v=u+gt$,
We have, $0=u sin\theta +(-g)t$
Or, $t=u sin\theta /g$ ………….(1)
Equation (1) is known as the time of maximum height.
Time of flight:
Now as the projectile reaches the ground after some time, the displacement along Y-direction is zero. If T is the total time taken by the projectile to reach B, then, we have
$s_{y}=$0. Using the equation of motion $s=ut+\frac{1}{2}gt^{2}$,
$0=T u sin\theta +1/2 (-g)T^{2}$
Or, $T(u sin\theta -gT/2)=0$
as $T\ne 0$
We have $T=2u sin\theta /g$ …………….. (2)
Equation (2) is known as time of flight.
Maximum height:
Now, if $H_{\max }$ is the maximum height of the projectile, then, using the equation $v^{2}$=$u^{2}+$$2gs$
We get, $0=u^{2}\sin ^{2}\theta -2gH_{\max }$
Or, $H_{\max }=u^{2}\sin ^{2}\theta /2g$ ……………… (3)
Equation (3) is known as maximum height of a projectile.
Horizontal range:
The horizontal distance travelled by the projectile from its initial position O to the position B is called the horizontal range, R.
As the horizontal range is along X-direction, we will consider the motion along X.
We have, $u_{x}=u cos\theta $ , $a_{x}=0$and $s_{x}=R=$horizontal range
Also, $T=2u sin\theta /g$
Using the equation of motion $s=ut+\frac{1}{2}gt^{2}$,
$R=u cos\theta (2u sin\theta /g)+1/2\times 0$
Or, $R=u^{2} 2sin\theta cos\theta /g$
Or, $R=u^{2} sin2\theta /g$ ……………… (4)
Equation (4) is known as the horizontal range of the projectile.
Equation of trajectory or path:
The equation of the trajectory of the projectile establishes a relationship between motion along the X and Y directions .
Now displacement (x) along the X direction is given by,
$x=t u cos\theta +1/2 \times 0$
Or, $t=x/ u cos\theta $ ……………(5)
displacement (y) along the Y direction is given by,
$y=t u sin\theta -1/2 gt^{2}$
Or, $y=(x/ u cos\theta ) u sin\theta -1/2 g(x/ u cos\theta )^{2}$, using the value of t from equation (5)
Or, $y=x tan\theta -$$\frac{1}{2}\frac{gx^{2}}{u^{2}\cos ^{2}\theta}$ ……………….. (6)
This is the equation of a parabola. Therefore, the path of a projectile is a parabola.
Equation (6) is known as the equation of trajectory.
