Square root of a complex number

The square root of x is the number r in a way that r² = x. When represented in form or specifically, , the square root of x might also be known as the surd or radical. Therefore, the square root is the nth root, having the value, n = 2.

It’s the point of notice that any real number which is positive contains 2 square roots in which one is positive and the other one is negative. Let’s say, the 16 is having square root as +4 & -4, since (-4)² = (+4)² = 16.

Any real number having non-negative value, x contains a unique square root which is non-negative which is ‘r’; and this is known as the principal square root & is written as, or .

Let’s say, the principal square root of 16 is √16 = +4, while the alternative sq. root of 16 = -√16 = -4. In general use, however, specified, the square root is usually taken into action which means as the “principal square root”. The function of the principal square root is ’s inverse function for the value, .

To Determine The Square Root Of The Complex Number a + ib

Where a &*b (≠0) are real values

Assuming √a + ib = x + iy, where value of x & y are for real.

Then, a + ib = (x + iy)² = x² + i²y² + 2x * iy = x² – y² + i *2xy ∵ (a +b)² = a² + 2ab + b²

Now, on equating both imaginary and real parts, we have,

x² – y² = a Eq. 1

2xy = b Eq. 2

Now, (x² + y²)2 = (x² – y²)2 + 4x²y² = a² + b²

Therefore, x² + y² = +√a² + b² (∵ x, y are both real) Eq. 3

Now, on the addition of equations – (1) and (3) we get,

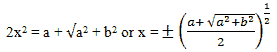
Now equation (3) – equation (1) gives,

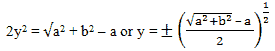
So, from the 2nd equation, it’s pretty much understandable that both the values of x & y consist of the same signs which can either be both +ve or both -ve.

When b holds the +ve value, and the values x & y encompasses two different signs, namely as the positive one and the other as the negative one. when b holds -ve value.

Therefore, if b > 0 then, as a result, you’ll see that the square roots of (a + ib) will be equal to:

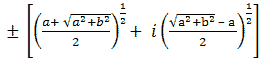
In the condition when b < 0, therefore, the square root value of (a + ib) will be in the form:

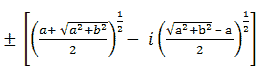
For further explanation, see a few of the examples:

Example 1:

Find the square root of 4 – 3i

Solution:

Let z² = (x + yi)² = 4 – 3i

Therefore, (x² – y²) + 2xyi = 4 – 3i

On comparison of real & imaginary parts,

x² – y² = 4 Eq. 1

2xy = -3 Eq. 2

Now, considering the modulus: |z|² = |z²|

x² + y² = Ö(4² + 3²) = 5 Eq. 3

On solving the two equations i.e. Eq 1 and Eq 3, we get,

x² = 2; y² = ½

Thus, x = ±√2; y = ±√1/2

So, from equation 2, x & y contains opposite signs

(x=√2 ; y = -√1/2) & (x = -√2 ; y = √1/2)

Hence, z = ±(√2 – √1/2i)