Stoke’s law is the basis of the falling-sphere measuring instrument, in which the fluid is stationary in a very vertical glass tube. A sphere of well-known size and density is allowed to go up through the liquid. If properly selected, it reaches velocity, which may be measured by the time it takes to pass two marks on the tube. Electronic sensing is often used for opaque fluids. Knowing the velocity, the dimensions and density of the sphere, and also the density of the liquid, Stoke’s law is often used to calculate the viscosity of the fluid. A series of steel ball bearings of various diameters are usually utilized in the classic experiment to enhance the accuracy of the calculation. The college experiment uses glycerine or sirup because of the fluid, and also the technique is used industrially to examine the viscosity of fluids utilized in processes. Many college experiments usually involve varied the temperature and/or concentration of the substances utilized in order to demonstrate the effects this has on the viscosity. Industrial strategies include many various oils and polymer liquids like solutions.
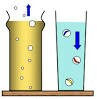
Stoke’s law is very important for understanding the swimming of microorganisms and sperm; additionally, the deposit of little particles and organisms in water, below the force of gravity.
In the air, a similar theory is often used to justify why little water droplets (or ice crystals) will remain suspended in air (as clouds) till they grow to a crucial size and begin falling as rain (or snow and hail). The similar use of the equation is often made in the settlement of fine particles in water or alternative fluids.
Stoke’s law assumption
Stoke’s law makes the following assumptions for the behavior of a particle during a fluid:
- Laminar flow
- Spherical particles
- Homogeneous (uniform in composition) material
- Smooth surfaces
- Particles don’t interfere with one another.
For molecules, Stoke’s law is used to outline their Stokes radius.
Flow around a sphere
For the case of a sphere in a very uniform so much field flow, it’s advantageous to use a cylindrical organization (r, φ, z ). The z-axis is through the center of the sphere and aligned with the mean flow direction, whereas r is that the radius as measured perpendicular to the z-axis. The origin is at the sphere center. As a result of the flow is axisymmetric around the z-axis, it’s freelance of the angle φ.
